📝题目
1 | 给定一个正整数数组 A,如果 A 的某个子数组中不同整数的个数恰好为 K,则称 A 的这个连续、不一定独立的子数组为好子数组。 |
📝思路
要说这题解是真的巧妙,精髓就在下图:“恰好”不好整,给整个“最多”,一下就变成老套路了——直接滑。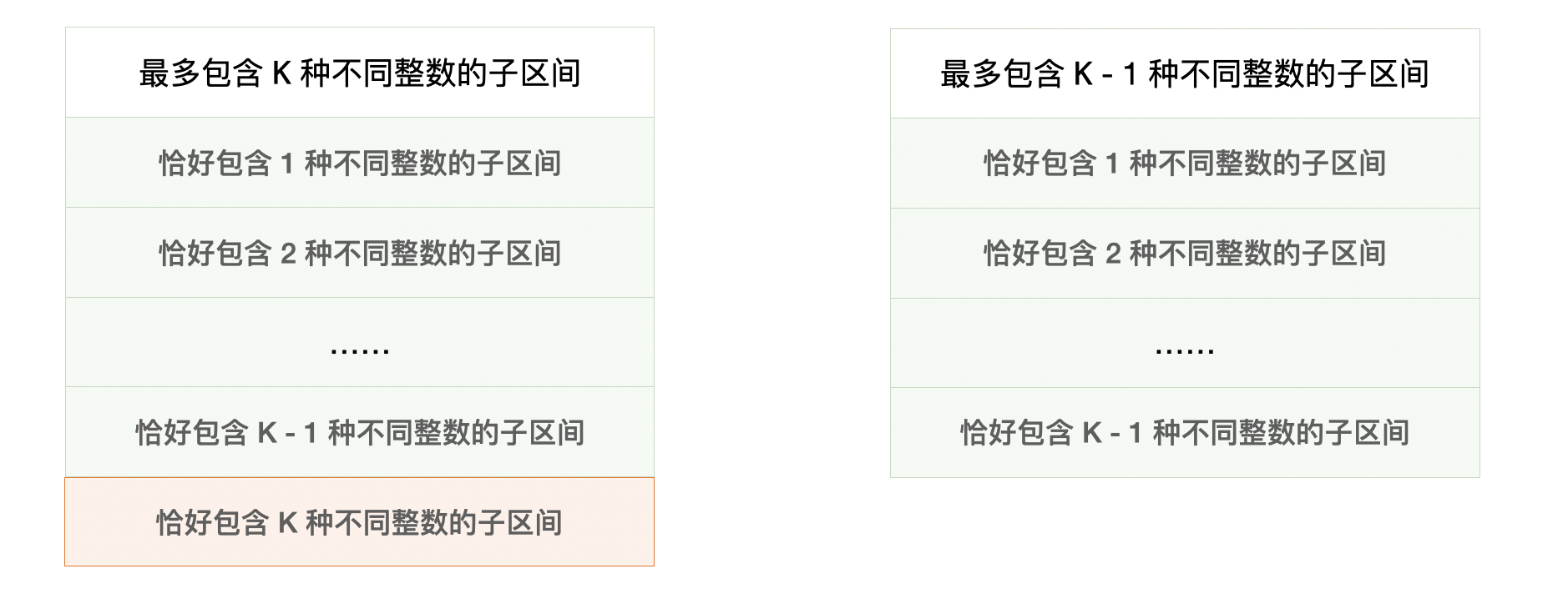
📝题解
1 | int subarraysWithMostKDistinct(vector<int>& A, int K) { |
All articles in this blog are licensed under CC BY-NC-SA 4.0 unless stating additionally.